A Variant of the Previous Game.
The game presented in the last section was studied by our club some years ago, and the result was published a well known journal Mathematical Gazette. In this year, we studied this game again, and tried to change the conditions of the game. We were eager to know if we get a Pascal-like triangle with a changed conditions. Fortunately, we discovered a new game that produce a Pascal-like triangle, and we are going to present it.
Game 1. There are p players, where p is a natural number. The game starts with Player 1 who takes out s cards, where s is a natural number. Then Player 2, 3,... ,Player p does the same. This is the first cycle. Then Player 1 starts again in the second cycle. This game ends when one of the player takes out a red card and becomes a loser.
The difference between this game and the original game is the number of cards players takes out at each turn. In the original game, each player takes out one card in each turn, but in this variant each player takes out s cards, where s is a natural number. For example, if s = 3, then each player takes out three cards in his or her turn.
The following Pascal-like triangle is mede by a game with p=3,s=3, where there are three players who picks up three cards in each turn.
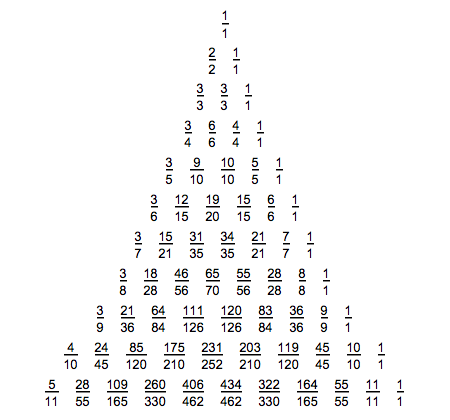
This new variant turns out to be a game that produces Pascal-like triangle. Therefore, the number of cards that each player takes out in his or her turn can be any number for a game to make Pascal-like triange.
Next, we change the number of cards each player takes out in his or her turn again.
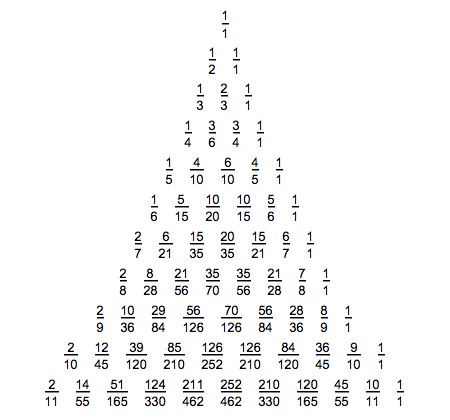
For an example, we present a game for three players. Player 1 takes out a card, Player 2 takes out two cards and Player 3 takes out three cards in his or her turn. Even this game produces Pascal-like triangle.(The following Figure presents probabilities of first player's losing the game.)
In fact, we have the following theorem.
There are players. Player 1, Player 2, ...,Player p takes out s1,s2...sp cards respectively. The game ends when one of the players takes out a red card in his or her turn, and this player becomes the loser. The probabilities of any player's becoming the loser make a Pascal-like triangle.
Here, numbers p and s can be any natural number for probabilities form a Pascal-like triangle. This is a generalization of the original game, and generalization is a very important method to study mathematics.
First, please choose numbers p, s and v, where p is the number of players, s is the number of times each player picks up cards and v is the order of the player. Then, please make a Pascal-like triangle using the following "Pascal-like Triangel Generator".
We use "pascal-like triangle gerator".
Game 2. : Two players take turns, and take out s cards, if he or she got a red card, the card is stored for him or her. Then, a player becomes a loser when the player takes out a red card and the total number of cards for him or her equals to g, where g is a fized natural number.
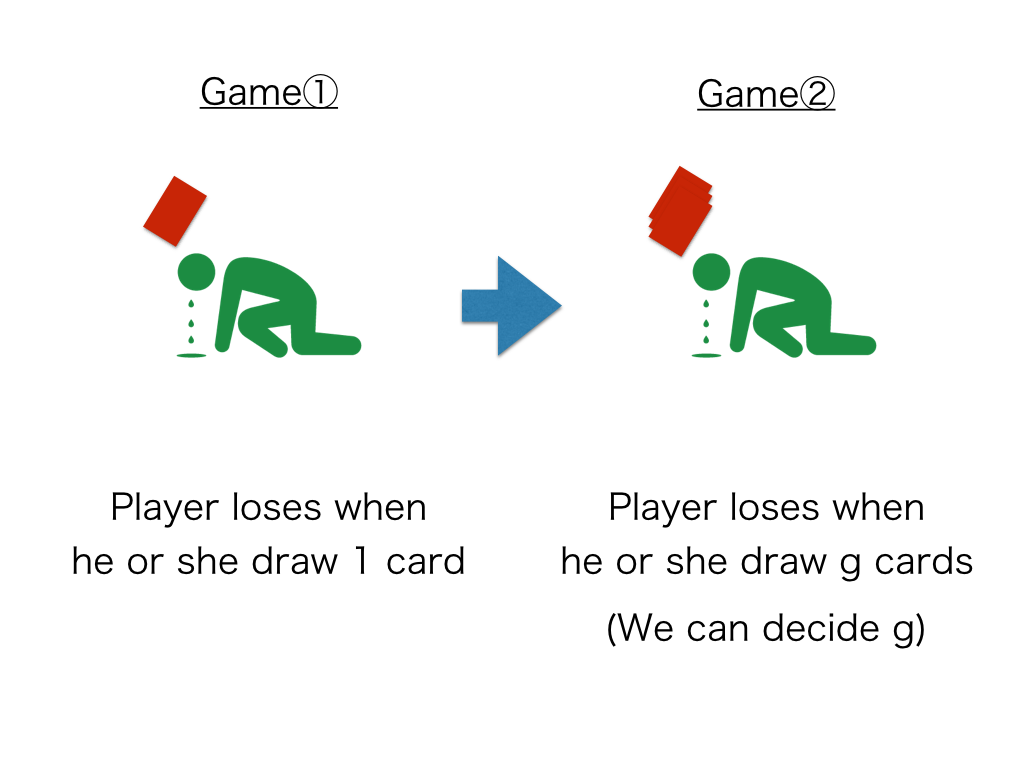
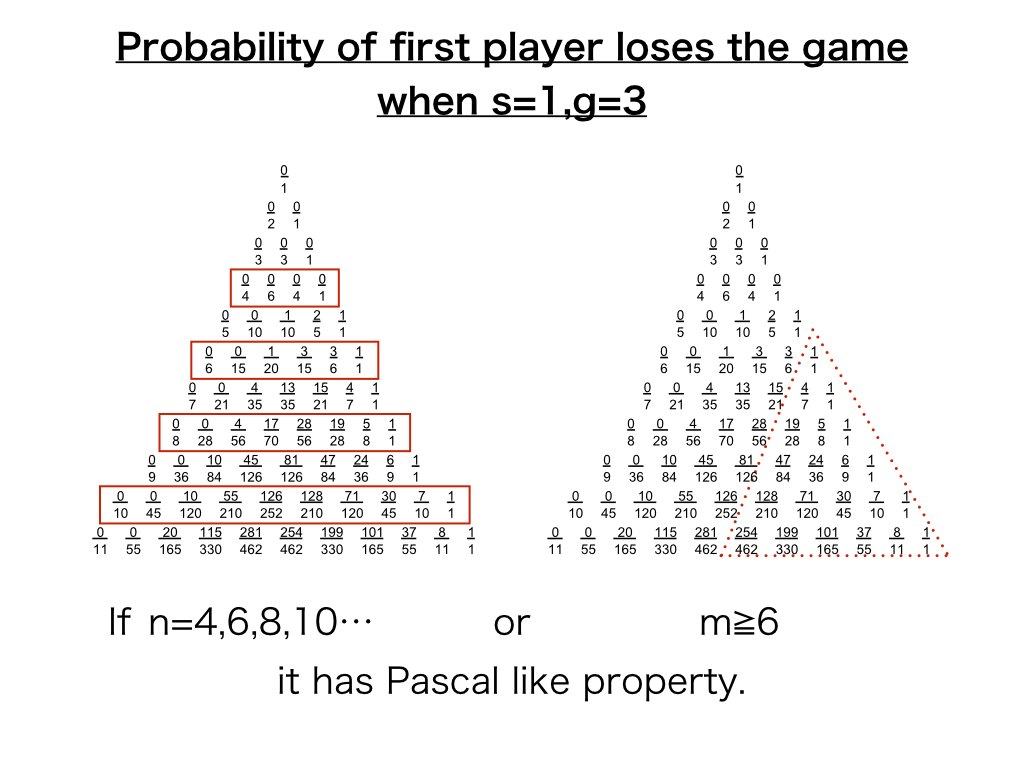
Note that we change the rule of losing the game. In previous games, if you choose a red card, you lose the game. In this new game, you lose the game when you collect a certain number of red cards. For an example, let g=3, then a player loses the game when he or she takes out a red card and he or she has already stored 2 red cards.
In this game Pascal-like properties can be found in a large part of the triangle of probabilities, but in some part you find the violation of the property. The fractions enclosed in a red lines satisfy the Pascal-like properties.
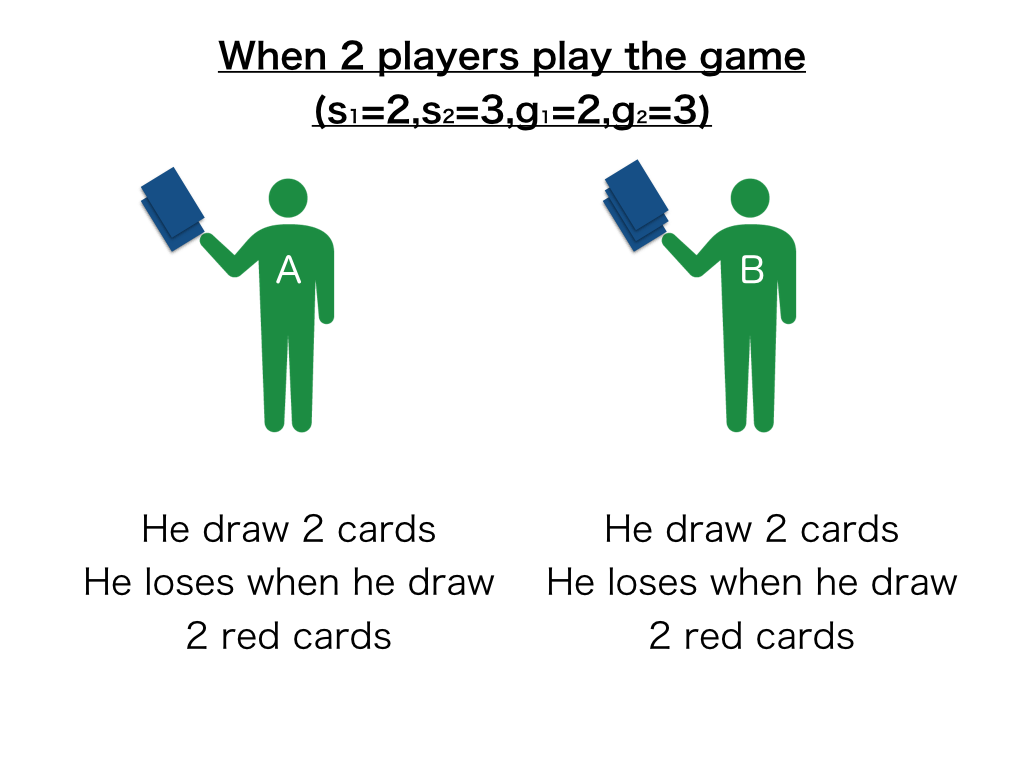
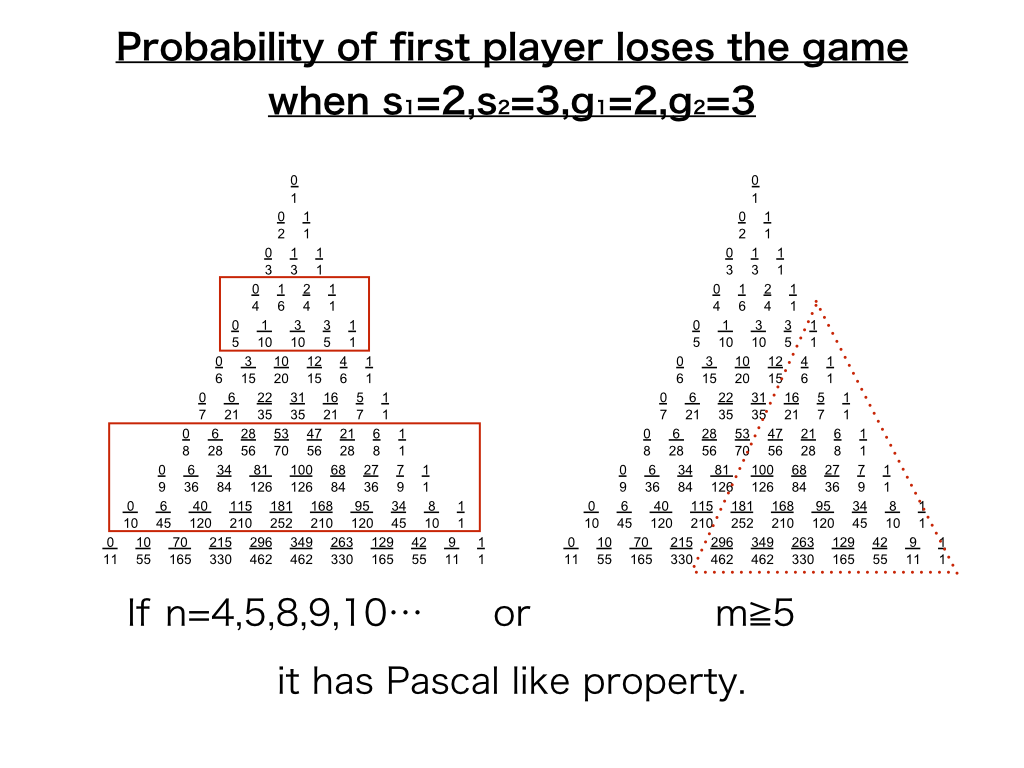
Next we change the rule of losing the game again, and each player has his or her own goal g of losing the game.
We also have Pascal-like properties on the places enclosed by red lines. In other part, we do not have the propeties.
Theorem
Two players take turns, and Player 1 and Player 2 take out s1,s2 cards respectivley. Player 1 loses the game when he or she collects g1, and Player 2 loses when he or she collects g2 . This game produces Pascal-like triangle when there is no draw.
Please choose variables s1,s2,g1,g2, and make a Pascal-like triange.
We use "Pascal-like Triangle Generator II".
So far, we have presented many kind of games that produce Pascal-like triangles.
