4.2 A Necessary Condition for a Chocolate Bar to have the Grundy Number 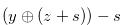 .
.
In this subsection, we study a necessary condition for a chocolate bar to have the Grundy number 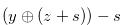 .
.
Definition 4.2. Let 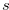 be a fixed natural number and
be a fixed natural number and 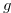 be a function that satisfies the following three conditions:
be a function that satisfies the following three conditions:
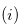
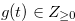 for
for 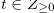 .
.
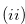
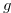 is monotonically increasing.
is monotonically increasing.
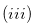 The Grundy number of
The Grundy number of 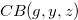 is
is 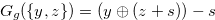 .
.
We are going to show that there exists a function 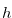 such that
such that 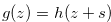 for any
for any 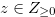 ,
,
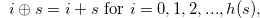 |
and the Grundy number of 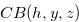 is
is 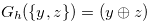 .
.
Lemma 4.6. Let 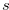 be a natural number and
be a natural number and 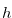 a function such that the conditions of Definition 4.2 are satisfied. Then we have
a function such that the conditions of Definition 4.2 are satisfied. Then we have 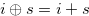 for
for 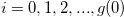 .
.
Proof. First, we prove that
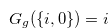 |
(4.20) |
for 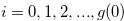 by mathematical induction. By the definition of Grundy number,
by mathematical induction. By the definition of Grundy number, 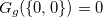 . We suppose that
. We suppose that 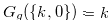 for
for 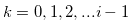 and
and 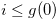 . By the definition of Grundy number,
. By the definition of Grundy number, 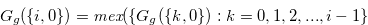
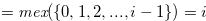 . By the conditions of Definition 4.2, we have
. By the conditions of Definition 4.2, we have 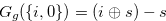 , and hence Equation (4.20) implies
, and hence Equation (4.20) implies 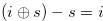 . Therefore, we have completed the proof.
. Therefore, we have completed the proof.
Theorem 4.2. Let 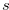 be a natural number and
be a natural number and 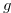 a function such that the conditions of Definition 4.2 are satisfied. We define a function
a function such that the conditions of Definition 4.2 are satisfied. We define a function 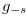 by
by 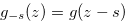 for
for 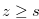 and
and 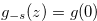 for
for 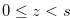 . Let
. Let 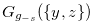 be the Grundy number of
be the Grundy number of 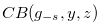 . Then
. Then 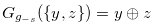 for any
for any 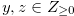 such that
such that 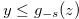 .
.
Proof. Case 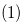 By the definition of
By the definition of 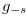 , we have
, we have 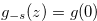 for
for 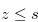 , and hence the function
, and hence the function 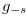 satisfies the condition of Definition 3.2 for
satisfies the condition of Definition 3.2 for 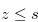 . Therefore
. Therefore 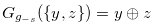 for any
for any 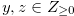 such that
such that 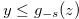 and
and 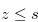 .
.
Case 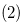 Next we prove that
Next we prove that 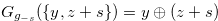 for
for 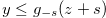 . We prove by mathematical induction, and we assume that
. We prove by mathematical induction, and we assume that 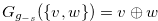 for
for 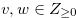 such that
such that 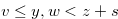 or
or 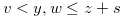 . By Lemma 4.2 and Lemma 4.6, we have Relation (4.21).
. By Lemma 4.2 and Lemma 4.6, we have Relation (4.21).
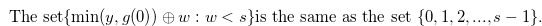 |
(4.21) |
By Definition 4.2,
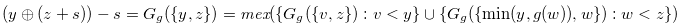 |
|||
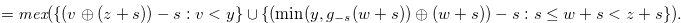 |
(4.22) |
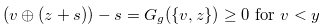 |
|||
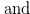 |
|||
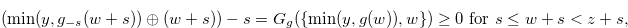 |
and hence we have
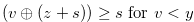 |
(4.23) | ||
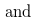 |
|||
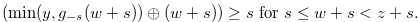 |
(4.24) |
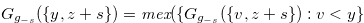 |
|||
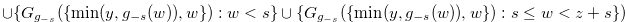 |
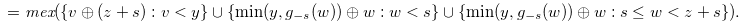 |
(4.25) |
Since 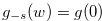 for
for 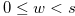 , Relation (4.21) implies
, Relation (4.21) implies 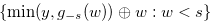
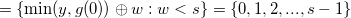 .
.
Hence, Equation (4.22), the inequality in (4.23), the inequality in (4.24), Equation (4.25) and Lemma4.3 imply 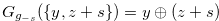 .
.
Theorem 4.1 and Theorem 4.2 prove the following proposition  and
and  respectively.
respectively.
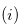 Let
Let 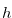 be a function such that the Grundy number of the chocolate bar
be a function such that the Grundy number of the chocolate bar 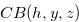 is
is 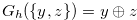 . Then the Grundy number of the chocolate bar
. Then the Grundy number of the chocolate bar 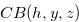 is
is 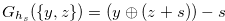 , where
, where 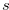 satisfies the condition (4.18) and
satisfies the condition (4.18) and 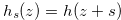 .
.
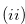 Let
Let 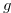 be a function such that the Grundy number of the chocolate bar
be a function such that the Grundy number of the chocolate bar 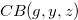 is
is 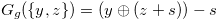 . Then the Grundy number of the chocolate bar
. Then the Grundy number of the chocolate bar 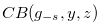 is
is 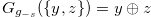 , where
, where 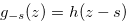 . Note that
. Note that 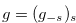 .
.
Therefore we have a necessary and sufficient condition for the chocolate bar 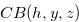 to have the Grundy number
to have the Grundy number 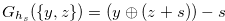 .
.
Next an example of this condition is presented for the function 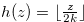 . As you see, this condition is quite simple for this function.
. As you see, this condition is quite simple for this function.
Corollary 4.1. Let 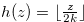 for a fixed natural number
for a fixed natural number 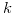 . Then
. Then
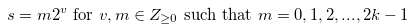 |
(4.26) |
if and only if the Grundy number of 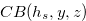 is
is 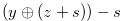 , where
, where 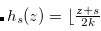 .
.
Proof. By Lemma 3.1, the function 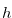 satisfies the conditions of Definition 3.2. By Lemma 4.1,
satisfies the conditions of Definition 3.2. By Lemma 4.1,
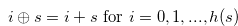 |
if and only if there exists 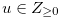 such that
such that
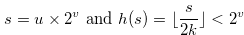 |
if and only if Condition (4.26) is valid. Therefore by Theorem 4.1 we finish the proof of this corollary.
- [1]
-
S. Nakamura and R. Miyadera, Impartial Chocolate Bar Games, Integers Volume 15, 2015.
- [2]
-
A.C.Robin, A poisoned chocolate problem, Problem corner, The Mathematical Gazette Vol. 73, No. 466 (Dec., 1989), pp. 341-343. An Answer for the above problem is in Vol. 74, No. 468, June 1990, pp. 171-173.
- [3]
-
D.Zeilberger, Three-Rowed CHOMP, Adv. Applied Math Vol. 26 (2001), pp. 168-179.
- [4]
-
M. H. Albert, R. J. Nowakowski and D. Wolfe, Lessons In Play, A K Peters, p-139.
- [5]
-
A.N.Siegel, Combinatorial Game Theory (Graduate Studies in Mathematics), American Mathematical Society (2013).